Magnus effect
The Magnus effect is the commonly observed effect in which a spinning ball (or cylinder) curves away from its principal flight path. It is important in many ball sports. It affects spinning missiles, and has some engineering uses, for instance in the design of rotor ships andFlettner aeroplanes.
In terms of ball games, topspin is defined as spin about an axis perpendicular to the direction of travel, where the top surface of the ball is moving forward with the spin. Under the Magnus effect, topspin produces a downward swerve of a moving ball, greater than would be produced by gravity alone, and backspin has the opposite effect. Likewise side-spin causes swerve to either side as seen during some baseball pitches, e.g. slider. The overall behaviour is similar to that around an aerofoil (see lift force) with a circulation which is generated by the mechanical rotation, rather than by airfoil action.
The Magnus effect is named after Gustav Magnus, the German physicist who investigated it. The force on a rotating cylinder is known asKutta–Joukowski lift, after Martin Wilhelm Kutta and Nikolai Zhukovsky (or Joukowski), who first analyzed the effect
A valid intuitive understanding of the phenomenon is possible, beginning with the fact that, by conservation of momentum, the deflective force on the body is no more or less than a reaction to the deflection that the body imposes on the air-flow. The body "pushes" the air down, and vice versa. As a particular case, a lifting force is accompanied by a downward deflection of the air-flow. It is an angular deflection in the fluid flow, aft of the body.
In fact there are several ways in which the rotation might cause such a deflection. By far the best way to know what actually happens in typical cases is by wind tunnelexperiments. Lyman Briggs made a definitive wind tunnel study of the Magnus effect on baseballs, and others have produced interesting images of the effect.The studies show a turbulent wake behind the spinning ball. The wake is to be expected and is the cause of aerodynamic drag. However there is a noticeable angular deflection in the wake and the deflection is in the direction of the spin.
The process by which a turbulent wake develops aft of a body in an air-flow is complex but well-studied in aerodynamics. It is found that the thin boundary layer detaches itself ("flow separation") from the body at some point and this is where the wake begins to develop. The boundary layer itself may be turbulent or not; this has a significant effect on the wake formation. Quite small variations in the surface conditions of the body can influence the onset of wake formation and thereby have a marked effect on the downstream flow pattern. The influence of the body's rotation is of this kind.
It is said that Magnus himself wrongly postulated a theoretical effect with laminar flow due to skin friction and viscosity as the cause of the Magnus effect. Such effects are physically possible but slight in comparison to what is produced in the Magnus effect proper. In some circumstances the causes of the Magnus effect can produce a deflection opposite to that of the Magnus effect.
The diagram at the head of this article shows lift being produced on a back-spinning ball. The wake and trailing air-flow have been deflected downwards. The boundary layer motion is more violent at the underside of the ball where the spinning movement of the ball's surface is forward and reinforces the effect of the ball's translational movement. The boundary layer generates wake turbulence after a short interval.
On a cylinder, the force due to rotation is known as Kutta–Joukowski lift. It can be analysed in terms of the vortex produced by rotation. The lift on the cylinder per unit length, F/L, is the product of the velocity, v (in metres / second), the density of the fluid,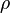 
(in kg / m3), and the strength of the vortex that is established by the rotation, G:
(in kg / m3), and the strength of the vortex that is established by the rotation, G:
where the vortex strength is given by
where s is the rotation of the cylinder (in revolutions / second), ω is the angular velocity of spin of the cylinder (in radians / second) and r is the radius of the cylinder (in metres).
1° CASE: Trajectory of a ball moving without rotationCASE: Trajectory of a ball moving without rotation
When the ball is kicked, the air adheres to its surface in the shape of thin concentric air layers.
When the air layer that is closest to the ball (limit layer) reaches the rear part of the ball, it is forced to detach, thus creating a series of vortexes behind the ball.
When the ball is kicked, the air adheres to its surface in the shape of thin concentric air layers.
When the air layer that is closest to the ball (limit layer) reaches the rear part of the ball, it is forced to detach, thus creating a series of vortexes behind the ball.
V = Speed of progress
If the ball is kicked without rotation (as in the picture below), the air flow around the surface is symmetrical and the air pressure is equal on the upper face and the lower face of the ball.
The result of pressures is VOID, so the ball trajectory is determined by the propulsive force (kick) on the one hand and the force of gravity and the forces of friction on the other hand.
The result of pressures is VOID, so the ball trajectory is determined by the propulsive force (kick) on the one hand and the force of gravity and the forces of friction on the other hand.
P = Air pressure
V = Speed of progress
V = Speed of progress
Regular trajectories are obtained, with a bend only on the vertical axis due to the force of gravity. In the powerful and close-range shots, the influence of the force of gravity is so low with respect to the propulsive force that the shots are basically straight.(According to the Bernoulli principle, the pressure exerted by a gas (air) on the surface of an object is inversely proportional to the speed the the gas itself on the surface: high speed = low pressure and low speed = high pressure.)
That is how EuroGoal reproduces a powerful shot without rotation: the trajectory is basically straight and very precise.
That is how EuroGoal reproduces a powerful shot without rotation: the trajectory is basically straight and very precise.
2° CASE: Trajectory of a ball moving with rotationIf the ball is kicked producing a rotation, its behavior changes radically and spectacularly: the air at its side which moves forward is dragged longer along the surface of the ball itself and detaches later.
The air that is on the opposite side detaches earlier.
The air that is on the opposite side detaches earlier.
The ball rotates and progresses through the air at the same time, so different interactions are created between the progress air flow and the concentric flows produced by rotation.
where flows have the same direction, air speed on the ball surface increases, while where they have opposite directions, they are in contrast and the air speed on the ball surface diminishes.According to the Bernoulli principle, air pressure on the ball is lower on the side where air flow quickly and higher where it flows more slowly.
The result of these two pressures is an F force (blue arrow) that augments the arching of the trajectory: this phenomenon is called Magnus effect.
where flows have the same direction, air speed on the ball surface increases, while where they have opposite directions, they are in contrast and the air speed on the ball surface diminishes.According to the Bernoulli principle, air pressure on the ball is lower on the side where air flow quickly and higher where it flows more slowly.
The result of these two pressures is an F force (blue arrow) that augments the arching of the trajectory: this phenomenon is called Magnus effect.
P = Air pressure on the ball surface
F = Force resulting from the pressure difference
F = Force resulting from the pressure difference
MAGNUS EFFECT APPLIED IN A PLAY SITUATIONIn real play situations, Magnus effect is used numerous times. The most spectacular are those regarding free kicks with wall.
The Magnus effect produces different trajectories according to the more or less inclined rotation axis.
Let us analyze two different situations in which EuroGoal perfectly simulates this kind of trajectories in order to create play situations that are particularly good for training.
The Magnus effect produces different trajectories according to the more or less inclined rotation axis.
Let us analyze two different situations in which EuroGoal perfectly simulates this kind of trajectories in order to create play situations that are particularly good for training.
- Shot with horizontal or slightly inclined wheels (max. 15°)The aim is to increase the ball arching on the horizontal axis to reach a target while avoiding opponents.
As can be seen, the ball travels along a trajectory that tends to go back towards the goal.
- Shot with inclined wheels from 15° to 80°. The aim is to increase the ball arching mainly on the vertical axis to go over the wall and reach the goal.
This kind of trajectory is used in volleyball, tennis and other sports, where it is called TOP SPIN.
This kind of trajectory is used in volleyball, tennis and other sports, where it is called TOP SPIN.
No comments:
Post a Comment